Back to the Astro-Mathematical
puzzle
Back to the description of the
complex fractal
The center of the self-exponentiated
fractal
A detailed look at the region near k=2 ball is
shown below.
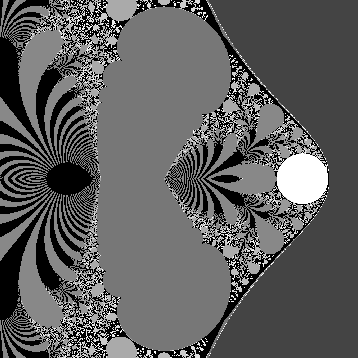
This is a central part of the "self-exponentiated"
fractal. The figure shows period k of the limit cycle of
...zzz.
The horizontal axis is Re(z) from
-0.85 to 0.15.
The vertical axis is Im(z) -0.5
to 0.5.
black: k=0 (or >40)
white: k=2
dark grey: k=1
lighter grey: k>4
A different coloring can be used on the above picture,
to highlight the relation between the k=5,6,7,8, and 9 regions (from blue-gray
to red), and thus illustrate the process of fractal leaf sprouting .

This is a central part of the "self-exponentiated"
fractal.
Black denotes k>9 or the divergence
of iteration. Lighter shade corresponds to higher k (from 5 to 9).
The traditional positioning of the complex plane is used,
with Re(z) extending horizontally and Im(z) vertically. To the right of
the k=2 ball (shaded white for better contrast) there is a stable
convergence region k=1 (binoculars, to the right of the white/red ball),
whose boundary is lined with a very thin stripe of k=2,
and probably also all higher values (too
thin to be resolved here). In other words, at least in that area, the loss
of stability of the fixed point of iteration happens trough period doubling.
Back to the home
page of Pawel Artymowicz
Back to the Astro-Mathematical
puzzle
Back to the description of the
self-exponentiated fractal


