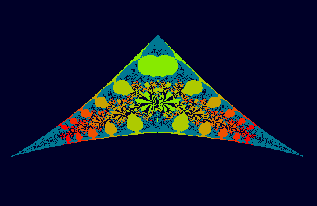
A detached ""trangular island" of a "self-exponentiated" fractal. The figure shows the period k of the limit cycle of ...z^z^z.
A detailed look at the region near z=-4.1, which can barely be seen in the more global views of the self-exponentiated fractal. This is probably the last fractal island of diverging exponentiation that lies at and near the real axis, as we move from -3 to -infinity. The surrounding sea has limit cycle period k=3.
A peculiar feature of this fractal island is that periodicities smaller than k=21 are not seen (at this resolution, but maybe at all: the only questionable site is the very edge of the island.) Starting from k=24 through 40, we have colored the appropriate regions with increasingly light grey. We have shaded k > 41 as very dark grey - these unresolved periods fill the trianglular region you see. Black is the divergence (k=0). The fractal leaf sprouting is clearly seen. In addition, many areas do have the shape of a leaf! The central "crab" has k=25.

A detached ""trangular island"
of a
"self-exponentiated" fractal. The figure shows the period k
of the limit cycle of ...z^z^z.
The vertical axis is Re(z) from roughly -4.16 (bottom) to 4.04 (top).
The horizontal axis is Im(z) -0.07
to 0.07.
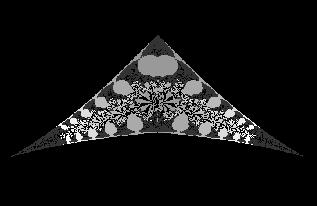
And this is the view of the fractal set where exponentiation diverges.

Black denotes k==0.