table of contents next topic

3. Massive planets and superplanets

As a reminder, tm is the mass doubling timescale of the planet. When given in unints of yr, Jupiter's orbital distance is assumed. Notice that closer-in planets will grow much more rapidly, in proportion to orbital period.

Model 46: 1 Jupiter-mass protoplanet in a standard nebula

  • Planet:
    		• mu = 10-3 e = 0
  • Disk:
    		•  alpha = 0.004 c/vK = 0.05
  • grid (phi,r): 278 x 220
    		• resol.: 0.0080 x 0.0048 tmax= 72 P
  • Accretion:
    		• 0.16*(dm/dt)dsk tm =
  • Migration (by gravity):
    		• -6· 10-5 vK (inward) ta= 31 kyr
  • Migration (by advection):
    		• +1.3· 10-7 vK (outward) ta= 14 Myr
  • Eccentricity:
    		• const. -

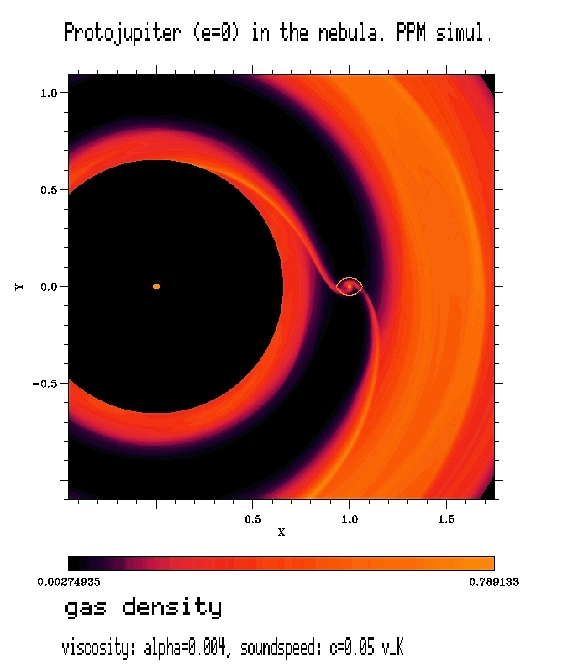
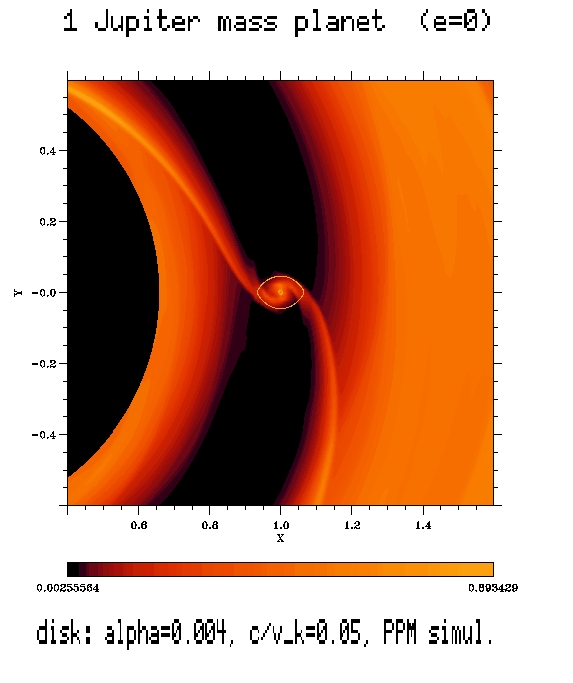
    Comments:
    Gap is open in agreement with all kinds of criteria.
    Mass flows through the gap. The accretion rate is intermediate. The boundary conditions inside the Roche lobe are such that only 3% of the mass is removed per dynamical time scale of the planet (or P/2/pi) inside the radius 0.6 rL from the planet. The actual accretion may proceed faster, depending on circumplanetary disk properties. Here, some pressure gradient builds inside the simulated Roche lobe and moderates the accretion, which may be unrealistic. The next model deals with this problem by assuming a faster removal of gas.
    Spiral shocks waves are seen in the gap, and continue as planet's wake into the disk forming nonlinear acoustic waves.

    Models 62,16,17: 1 Jupiter-mass protoplanet in a standard/thick solar nebula

  • Planet:
    		• mas ratio µ = 10-5 e = 0.01
  • Disk:
    		•  alpha = 0.008 c/vK = 0.025
  • Grid (phi,r): 176 x 140
    		• resol.: 0.013 x 0.0012 tmax= 140 P
  • Unpert. disk accretion:
    		• (dm/dt)dsk=3 pi Sigma nu = 3.2· 10-8 Msun/yr
  • Accretion:
    		• 1.3*(dm/dt)dsk tm = 24 kyr
  • Migration (by gravity):
    		• 2.0· 10-5 vK ta= 94 kyr
  • Eccentricity: damped
    		•  de2/dt=-2· 10-6 /tdyn te= ? yr

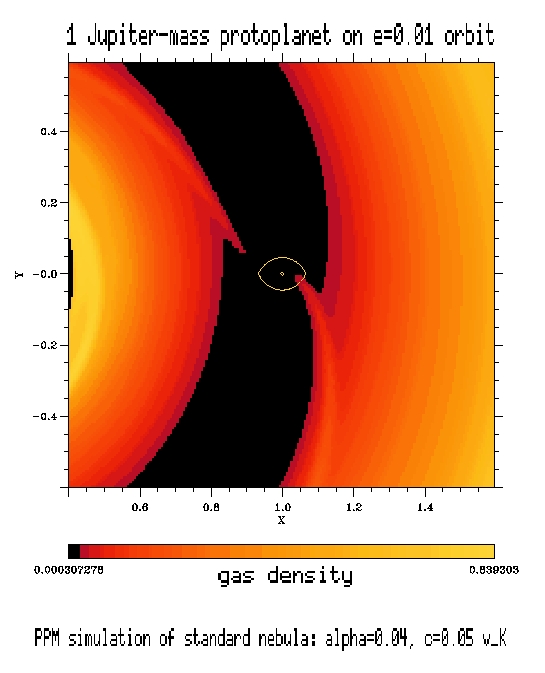
    Model 04: 3 Jupiter-mass protoplanet in a standard nebula

  • Planet:
    		• mass ratio µ = 3 · 10-3 e = 0.01
  • Disk:
    		•  alpha = 0.004 c/vK = 0.05
  • Grid (phi,r): 108 x 90
    		• resol.: 0.02 x 0.02 tmax= 100 P
  • Accretion:
    		•  dm/dt= 0.5 × (dm/dt)0 tremove = 0.02 P (max. efficient)
  • Unpert. disk accretion:
    		• (dm/dt)0 = 3 pi Sigma nu nu = 10-5
    - (dm/dt)0 = 6 · 10-8 Msun/yr -
  • Doubling time:
    		•  tm = 0.19 Myr !
  • Migration (by gravity):
    		• da/dt = -5.5 · 10-6 vK (inward) ta= 0.340 Myr
  • Migration (by advection):
    		• da/dt = +1.6 · 10-6 vK (outward) ta= 1.2 Myr , adv < grav (=> inward)
  • Unpert. disk flow:
    		• v = -1.5 · 10-5 vK tvisc= 0.13 Myr
  • Eccentricity (by gravity) :
    		• de2/dt = +-3 × 10-8 (noisy) grav = adv (?)
  • Eccentricity (by advection) :
    		• de2/dt = -3.2 × 10-8 te = 12 kyr
    table of contents