---

Work  in progress:

Accretion  of   gas  from  circumbinary  disks  onto  forming   binary  systems

    t = 93 P        box side = ~4.5 a                             t = 156 P           box side =  ~7 a

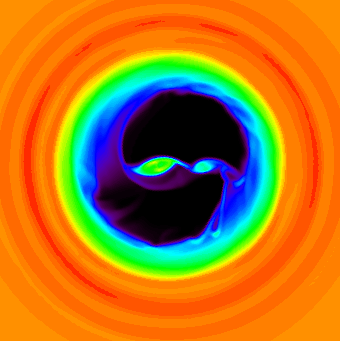
Why is this face in the sunglasses in the left panel smiling?
Because it's a snapshot from my first PPM hydrocode simulation of a binary system, accreting gas from a circumbinary disk, i.e. a disk around a double star (Piecewise Parabolic Method of Woodward and Collela, 1984; the code is based on John Blondin's VH-1). The sunglasses in the middle are, in fact, the (larger) circumprimary and the (smaller) circumsecondary `disks'. I hope that AMR (adaptive mesh refinement) and parallel power of Hydra (cf. note at the bottom) will soon help me resolve these regions better, which will allow to zoom in on the two point masses orbiting each other in the simulation.

The scale is logarithmic to show the low levels of gas density (in addition, there are density waves in the CB disk as seen on the right, at time t=93 orbital periods).  In the right-hand panel,  assuming that your monitor is set up to display the same color as mine, the orange color is disk density~1, green color seen in the circumstellar disks is for densities~0.01-0.1, and blueish-black colors for ~0.001 and lower densities (down to 5E-10).  This model has a temperature profile corresponding to z/r~0.05 at the disk's inner edge. The sizes of boxes are:   4.5a  and   7a, where   a = semi-major axis of the binary system. As you see,  the flow is not fully stationary, i.e., it does vary somewhat with time, even when viewed in the corotating frame where binary potential is stationary, because of a very small  level of numerical viscosity inherent in the PPM scheme  presumably unable to kill the initial transients (no artificial or Navier-Stokes viscosity has been used in this run).

With Steve Lubow, we have previously studied the time dependent streamers of gas falling  from a CB disk onto a binary with the mass parameter m2/(m1+m2)=0.3 (as in the above picture), based on low resolution SPH  models. This particular PPM hydrocode simulation traces the streamers much better (down to much lower densities, better resolution in the vicinity of stars). It also differs from our previous work on eccentric binaries, in that  we have here a circular orbit binary. The phenomenon of gas flow through gaps in accretion disks, which we discovered, is very common and robust,  and does not critically depend on the binary or disk parameters (it never switches off completely, for instance).

The models of the kind shown here are relevant to the question of origin of  large planets (superplanets) in extrasolar systems, binaries including brown dwarfs, and double stars,  the variability of the pre-main-sequence binaries (T Tau, UX Ori stars), and the double supermassive black holes in the nuclei of merging galaxies.